Financial IQ is critical to becoming successful and getting rich.

IRR is a calculation and a decision rule for project finance and investment.

Let’s explore using the internal rate of return as a capital budgeting tool for deciding how to best to invest and allocate money.

Internal Rate of Return, IRR, is similar to, and derivative from, NPV. It restates NPV as a percentage rate. It is a measure with intuitive appeal. It provides a way of grasping the rate of return a project, or income-producing asset, is yielding.

Internal rate of return (IRR) is the discount rate used in capital budgeting that makes the net present value of all cash flows from a particular project equal to zero.

The higher the internal rate of return of a proposed project, the more desirable it is to undertake the project.

The internal rate of return is derivative of NPV. NPV basically tells us whether or not the present value of the cash coming in exceeds the cash going out. NPV calculates the net of the present value of the cash flows. With IRR we come at the issue from a different angle.

First we compute an NPV and then we determine what discount rate sets the NPV equal to 0. This is the discount rate that makes the cash outflow equal to the present value of the cash inflows. Our decision rule is: to invest if that rate is bigger than our hurdle rate.

Internal rate of return is basically asking: how much do we need to discount the cash flows in order to drive the value out of the project? It’s a measure of resiliency of the cash flows.

The more we discount, the more that drives down the NPV. How much do we have to discount it and still stay bigger than 0? That’s what IRR is designed to tell us. This decision rule is very similar to our net present value decision, as we’ll see graphically down below.

Internal rate of return takes the net present value, which is a numerical value, and restates it as a percentage, which makes it more intuitively appealing.

It’s difficult for us to grasp the meaning of a number. It has little context. If I say the NPV is $3,537,000 it means little by itself. If I tell you the internal rate of return on the project is 25%, it’s easy to intuitively grasp. It takes the net present value and changes it into a percentage that’s easier to quickly grasp and understand.

IRR gives us a more intuitive and appealing take on the NPV of the project. IRR doesn’t tell the whole story though, and it’s best to always use it in conjunction with NPV.

IRR Formula

Let’s think about IRR in formulaic terms. In a previous post, when we had our net present value formula, the NPV was equal to the initial cost weighed against all of the cash flows coming in off the project in future years.

NPV = — Initial Costs + CF1/(1+r) + CF2/(1+r)2 + CF3/(1+r)3 ….

That was our formula for the NPV. For IRR, we’re going to take that exact same formula, the initial cost, and instead of using r the discount rate, we replace that with IRR and set that equal to 0.

The discount rate that sets this series of numbers equal to 0 is IRR.

0 = — Initial Costs + CF1/(1+IRR) + CF2/(1+IRR)2 + CF3/(1+IRR)3 ….

It’s really hard and incredibly time consuming to solve the polynomial equation for IRR with pen and paper, but it’s easy to do it in Excel or on a financial calculator. They have IRR functions built in. I prefer Excel or a spreadsheet program on a computer because you can build out the model and save the results. This is important if later your boss or client asks how you came up with that number. Always save your work for later reference.

Let’s look at the relationship between NPV and IRR graphically. Here we have a project that has a positive NPV and we graph the value of the net present value in dollars.

As we discount the cash flows with progressively higher and higher discount rates, the NPV is going to decline until, at some point, it crosses 0.

The point at which that crosses 0, the discount rate that sets the NPV equal to 0, is the IRR.

Any time the discount rate is below the IRR, it’s a positive NPV project. So if our hurdle rate is 7% and the IRR is 12% it’s a good project. IRR is similar to NPV, except that we have discounted the cash flows to a percentage rate where the discounting just crosses to negative, at 0.

Now we can say, for example, the return on this project, the IRR, is 12%. And that is easier to understand than saying the net present value is $1,613,672, which is just a big number with no context. We don’t really know what it means. But if the return is 12%, we can compare: 12% relative to a 7% cost of capital. That’s a good project. That’s what IRR does for us.

IRR Example

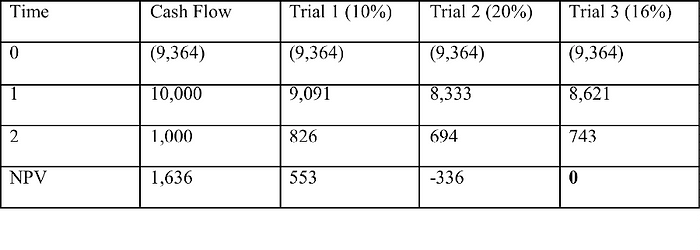
Let’s think this through in terms of an example. Let’s say I were spending $9,364 in order to generate cash of $10,000 in year one, and $1,000 in year two. If I didn’t do any discounting at all and just added up the cash flows that would be 1,636. 36 If I discounted the cash flows at 10%, I would discount the $10,000 back one period at 10%, discount the $1,000 back two periods at 10%, give me a net present value of 553. If I discount the cash flows at 20% instead of 10%, I get a net present value of minus 336.

So what must have happened somewhere in between? Somewhere that net present value crossed from positive to negative. If we solve it, that actually happens at exactly 16%. That’s the point where we are discounting it enough to draw all the value out of it.

In the above example we have to keep guessing and solving for different discount rates until we converge on an answer. IRR is a really easy thing to do in Excel. Built into Excel is an IRR function. There many tutorials online that will show you quickly how to use that function in your spreadsheet or financial calculator. It solves that polynomial equation, finds the root, sets it to 0, and gives us the solution.

Calculating IRR

There is no fancy formula for IRR where you can just plug in the numbers and calculate the solution. It can only be calculated via an iterative process of trial and error, with each successive iteration getting closer and finally converging on the solution. This type of convergence algorithm is what computers excel at.

Microsoft Excel does it for us by crunching through thousands of iterations in a fraction of a second. Before spreadsheets became ubiquitous on personal computers you could estimate IRR with cross-reference tables. Now with just a few mouse clicks, we get an accurate IRR solution.

Here is a short video to help solidify the IRR concepts and calculations. Its from my online corporate finance course.

IRR Summary

Internal Rate of Return, IRR, is similar to, and derivative from, NPV. It restates NPV as a percentage rate. It is a measure with intuitive appeal. It provides a way of grasping the rate of return a project, or income-producing asset, is yielding.

IRR accounts for the timing, the opportunity cost, and the risk of a project in a similar way to NPV. IRR is a good capital budgeting tool but it should not be relied upon as a standalone measure. We should always compute it alongside of the gold standard NPV.

Want More?

Check out my award winning finance book.

Skip Business School. Educate Yourself.

This story is published in The Startup, Medium’s largest entrepreneurship publication followed by + 375,985 people.

Subscribe to receive our top stories here.

‍